NavList:

George Huxtable wrote:

Three separate observations are made, one of each star. By our prior condition, the probability of each being toward or away is 50:50. There is no correlation between them. They could have been made by three separate observers, each putting his observation into a sealed envelope, to be opened together after the event. Then the probability of any combination, of the 8 possible, is one in 8. How can it be different?
--------------
Now I think we’ll back up a little. We’ll take the same approach that I used in my discussion of the “long-run” running fix – the Bayes (and E.T. Jaynes) logic of inference, used in the face of incomplete knowledge: Now say we’ve made a lot of measurements with the device that locates our LOPs and have found that random errors occur with a normal distribution of standard deviation, sigma.

Next we acquire three LOPs. They do not intersect at a common point. We don’t know where the true fix is. We do know that each LOP represents a sample from a normal distribution, each with the same sigma. Lacking any other information, we are forced to assume that each LOP locates the center of a linear normal distribution in the x-y plane (see the first attachment below). This is a very poor assumption, but it’s the best we can do. Are we to assume the LOPs are located somewhere less likely in their distributions? (Here we see a major difference with another logic that might start by considering the three probability distributions of LOPs intersecting at precisely the same point, the true fix location. But this contradicts the Bayesian logic because, in fact, we don’t know the true fix location. This might be Georges logic.)

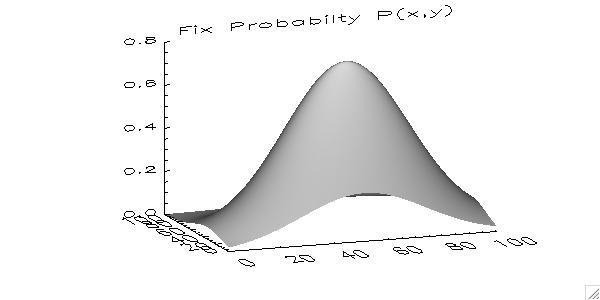
Using our logic, the three distributions are uncorrelated (in fact they are in disagreement). And the probability of point in the x-y plane being a fix from all three normal distributions is the product of the three distributions centered on the three LOPs. This gives a plot of the probability per unit area P(x,y) that the fix is at a given x-y point, as shown in the second attachment. We see from this figure that, because of the shape of the normal distribution, there is one single point of MP (maximum probability). The probability that the fix is inside any given area is the integral of P(x,y) over that area. (I’ve numerically calculated some of these probabilities, such as the 84% and 94% that I’ve posted earlier.) We see that areas of different shapes, different locations, and different sizes can have very different probabilities that the fix is inside them. This explains George’s question above, how different probabilities arise. George seems to be counting types of regions, rather then calculating the different probabilities that the fix is inside them.

Since P(x,y) is a probability density – a probability per unit area -- we don’t talk about the probability that the fix is at a specific point. That would be zero since a point encloses zero area. Rather we do calculate and plot the value of P(x,y) -- the density. Those are the values on the contours I’ve posted. For example a value of P = 0.013 means that the probability that the fix is inside a unit area surrounding P(x,y) is 1.3%. (The 100x100 plot, representing 10x10 nm had 100x100 data points. So the unit area is 0.1x0.1 nm.) The values of P(x,y) are small because the total area is so large, 10,000 data points.

It is true that as the cocked hat becomes smaller the MP inside it increases, but it never can exceed one, So the probability that the fix is inside the hat decreases to zero as the hat’s area decreases to zero. Thus the navigator’s “desired” result of three LOPs intersecting at one point means, that in all probability, the true fix is not there. But of course, the probability that the fix is somewhere in the x-y plane is always 100%. It follows that the probability that the fix is outside the hat is 100% minus the probability the fix is inside. Since the probability that the fix is inside goes to zero with the hat size going to zero, the probability that the fix is outside goes to 100%. There can be no constant 75% of the fix being outside.

Finally from considering the form of P(x,y), as I’ve point out, the location of the MP minimizes the sum of the squared distances to the three sides of the cocked hat. Now Herbert Prince (and Frank) tells us that this point is called the symmedian of the cocked hat, as explained by Villarceau in 1877. Have we navigators been lost all this time??

JK
----------------------------------------------------------------
NavList message boards and member settings: www.fer3.com/NavList
Members may optionally receive posts by email.
To cancel email delivery, send a message to NoMail[at]fer3.com
----------------------------------------------------------------