NavList:

Tony, I don't have a specific answer for you. Maybe Lars Bergman or someone else has reverse-engineered this method? I haven't spent any time on it for several reasons:

• In the late 19th century many "little methods" were published and each was proclaimed as the easiest, bestest, most wonderfullest method every proposed for clearing the lunar distance. Most of these were in fact re-hashes of well-known methods.
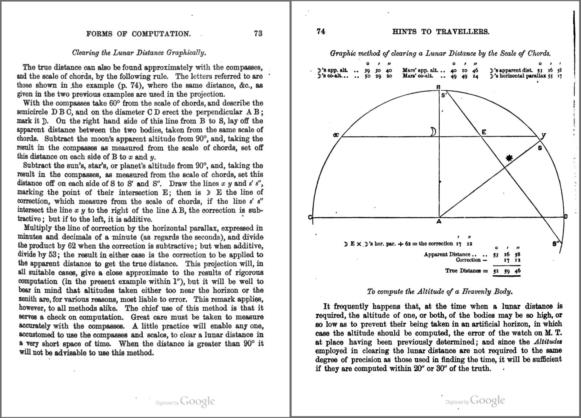
• This graphical method is described in Hints to Travellers in 1883 but not in other editions. There is a reason for that, yes?
• The article implies an accuracy which is almost certainly misleading when it says that the lunar example is accurate to one second of arc (this fits with my first point).
• This book includes a number of misleading and incorrect statements about lunars. It is roughly fifty years past the peak of regular maritime use of lunars and even past the period when lunars still had some value in land exploration. The subject was suffering from entropy.

If you want an approximate clearing of a lunar to get GMT, you can do it in a few very short steps with a calculator (or logarithms), and I suspect this graphical method is an implementation of the P₁ and P₂ part below:

• You pre-clear the lunar distance by adjusting the altitudes of the Moon and Sun (ALTm and ALTs) for IC, semi-diameter, and dip (if any) at a low accuracy level, and by correcting the LD for IC and adding the two semi-diameters to the lunar distance itself but with high accuracy (including augmentation of the Moon's SD). Subtract the Moon SD instead of adding for a "Far" limb lunar. Moon-Sun lunars are always "Near".
• Calculate P₁ = HP·sin(ALT_m) / tan(LD)
• Calculate P₂ = HP·sin(ALT_s) / sin(LD)
• Skip the lookup of a third correction: TC
• LD_c = LD + P₁ - P₂ + TC ; since we skipped it, TC is zero.
• Interpolate LD_c between known LD values (predicted lunar distances from an almanac or other database). Result is Greenwich time (GMT if the predicted distances are for Mean Time, GAT if the distances are listed for Apparent Time).

And that's it. You have cleared the lunar distance and determined Greenwich time ...approximately. I suspect that the graphical method is an implementation of the calculation of P₁ and P₂, but that's a guess. Limiting ourselves to altitudes above 20° and lunar distances above 20° the third correction that we skipped leads to errors in the clearing of around 1 or 2 minutes of arc but more for larger lunar distances. Every minute of arc error in clearing the distance corresponds to two minutes error in Greenwich time which implies 30' error in longitude. That can be acceptable for certain narrow purposes but clearly skipping TC is not a good idea!

Many standard series methods of clearing lunar distances were based on this short computation, and a variety of tables for the "third correction" were published including the well-known Thomson Tables. Note that P₁-P₂ is simply the "linear" part of the Moon's parallax in altitude that acts along the lunar arc while the third correction (TC) contains all the small details including refraction for both objects and "quadratic" corrections. When done right this process is slick. It is indeed the fastest, bestest, and easiest way to clear lunars. I think it's clear that the short calculator or logarithmic computation required to get P₁ and P₂ probably takes no more time --and quite possibly less time-- than the careful drawing of arcs and lines required in the graphical approach published in Hints to Travellers in 1883. 

Frank Reed

File: