NavList:
A Community Devoted to the Preservation and Practice of Celestial Navigation and Other Methods of Traditional Wayfinding
Certaine Errors in Navigation Corrected
From: Gary LaPook
Date: 2007 Sep 21, 13:29 -0700
In 1980 I discovered a book in my college's library
which was a reprint of Edward Wright's "Certaine Errors in Navigation"
which had been published in 1599. I still remember how pleasurable it
was to read, it was like sitting down with an old friend and discussing
navigation. He seemed like a thoroughly modern man. I highly recommend
this book if you can find a copy. There were other navigation books
reprinted as part of "The English Experience" series and they were also
worth reading.
Edward Wright was a mathematician who turned his interest to navigation and was the first one to devise how to compute the table of meridional parts necessary to create charts on "Mercator's Projection" and published such a chart covering England to the Azores based on a voyage he took with the Earle of Cumberland in 1589. I attached a copy of that chart to a previous post. For biographical information on Wright you can go to: http://books.google.com/books?id=uTsJAAAAIAAJ&pg=PA101&lpg=PA101&dq=certaine+errors+in+navigation&source=web&ots=GqTcQV1VCi&sig=lyxvKOsPG1jE98_lxYLsaXprEiA#PPA101,M1
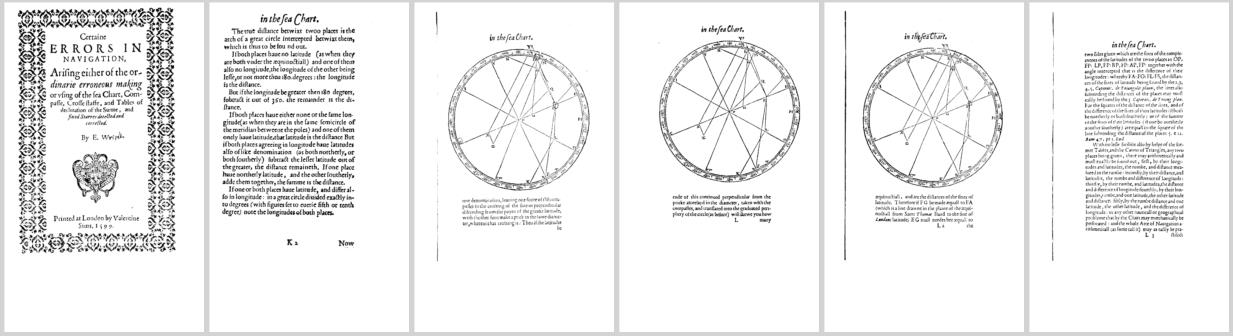
In "Certine Errors" Wright laid out a method to compute the great circle distance between two points on the earth using only a straight edge and a pair of dividers! I have never seen this method described in any other navigation text. Although it is now trivial to do this computation with a calculator it think it might be interesting to look at Wright's method. I am attaching 13 pages of his book that I photocopied back in 1980 but I am missing several pages which would fall between the second page of reproduced text (ending with "places.") and the next reproduced page starting with "foote," (they didn't number their pages.) Don't be intimidated by the type and spelling as you quickly get the hang of it and can read it as quickly as modern text. There also appears to be a couple of typographical errors that I will point out.
I will also offer my own explanation.
Wright starts out with special cases but I think it is easier if we start out with the general case. Wright's description of the general case starts on the last line of the third page of reproduced text ("If the latitudes be not both equall...") and continues with a couple of examples using London and Jerusalem ("Hierusalem") and London and Cusco as the examples. The coordinates he uses for London are 51º 32' north (which is as accurate as its modern latitude and a longitude of 22º (all of his longitudes are east). ( I do not know where he got this value since it does not comport with his own chart (which would make it about 16º) published in the same book, perhaps an earlier longitude using a different prime meridian (perhaps the Cape Verde Islands) since he couldn't be off by 6º between the Isle of Wright and London.) The latitude of Jerusalem he gives as 32º north while its modern value is 31º 16' north and he uses 11º south for Cusco while its correct value is 13º 28' south. The longitude he uses for Jerusalem is 68º, making it 46º east of London while its true value is 35º 14' east, a longitude error of 11º. He gives the longitude of Cusco as 295º making it 273º east of London while its modern longitude is 72º west, the same as 288º east of Greenwich showing an error of 15º between Wright's longitude and the modern value.
We will use Wright's values for this illustration, 51.5 for latitude of the London, 22 for the longitude of London; 32 and 68 for Jerusalem; and 11 south and 295 for Cusco.
Wright produces a diagram of the method however is is very complicated since all the lines for his many examples are contained on one diagram. We will draw only the lines we are interested in but will use Wright's labels for the lines.
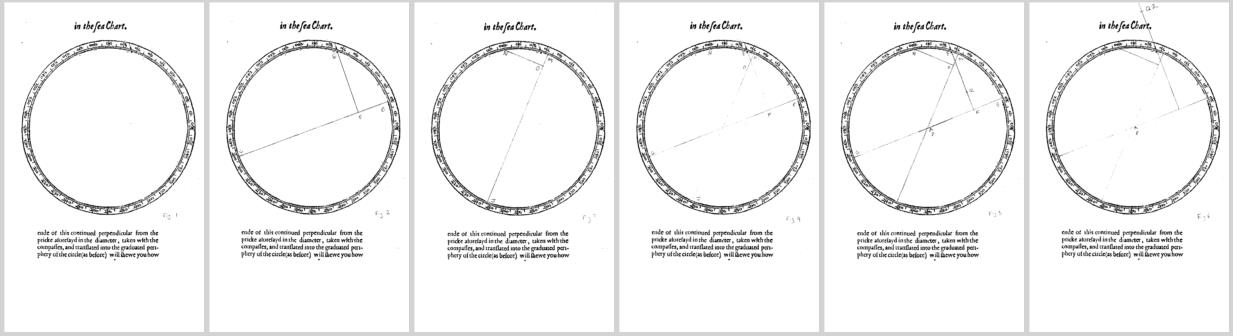
Starting with the first example of figuring out the distance between London and Jerusalem, we first draw a line across the base circle diagram from the longitude of London, 22, "B" all the way across to the opposite side at 202., "C." Wright would have us next do the same for the longitude of Jerusalem but instead lets draw the line representing the latitude of London. Add its latitude, 51.5, to 22 and place a dot on the circle at 73.5, "E." Then taking a triangle or a plotter place its straight edge on the line "B-C" and draw a line from "E" that is perpendicular to "B-C," marking the intersection as "F." (Of interest is that the length of "E-F" is the sine of 51.5 and the length from the center of the circle (not labeled) to "F" is the cosine of 51.5.)
Next we do the same for the position of Jerusalem, drawing a line from 68, "M," across to 248, "J." Then we draw the perpendicular representing Jerusalem's latitude, from 100 (68+32) "N" to intercept "M-J" at "O." (The lengths of these lines represent the sine and cosine of 32.)
Since London and Jerusalem are both in the northern hemisphere, we next subtract the length of Jerusalem's latitude, "N-O," (the smaller value) from the length of the latitude of London, "E-F," by taking a pair of dividers and setting them to "N-O" and then placing one point on "E" and the other point on the line "E-F" and marking this point "Q." (Note, if you are following Wright's description at this point, there is a typo in the text were it states "and P Q equall to N O" it should say "and E Q equall to N O.") Next we determine the distance between the points "O" and "F" (the intersections of the latitude lines with their respective longitude lines) with our dividers and leaving one point on "F" we swing the other point to the line "B-C" and mark it "P." Leaving that point on "P" we next move the other point of the dividers to "Q," this is the end of the chase. With the dividers set to the spacing between "P" and "Q" we move the dividers to the scale on the circle and take out the number of degrees between the divider points, this is the number of degrees of the great circle joining the two places on earth. Multiply by 60 to calculate the number of nautical miles.
Does it work? Wright came up with "38 degrees and about 3/4 that is 775 leagues, which are 2325 miles." Using a modern digital calculator your end up with 38.614 degrees, 2316.8 miles a difference of 8.2 miles or a 0.3% difference. Not bad with just a straight edge and dividers.
Wright uses London and Cusco to illustrate the case when the two places are on opposite sides of the equator. The only difference is that instead of subtracting the latitude of one from the other you add them. You do this by extending the longer latitude line till it goes outside of the circle and then marking on this extended line the distance of the latitude of the other location. Using the diagram we already have and pretending that Jerusalem's latitude is south instead of north, we extend the line "E-F' some convenient distance outside the circle. Then placing the divider points on "N-O" we take off that spacing and placing one point on "E" we place the other point on the extended line outside the circle and this becomes our new "Q," lets call it "Q2." Then taking the distance from "Q2" to our original "P" we take out the spacing and place the dividers on the circle and find the number of degrees of the great circle between London and a Jerusalem located in the southern hemisphere. I come up with 92 degrees for a great circle distance of 5520 miles. Using a computer I get 5565 miles, a difference 45 miles or 0.8%.
Wright uses Cusco to illustrate this case, drawing in lines "R-V" and "S-T" in addition to the original lines for London. Taking the distance between "S" and "F" and using it to establish "X, "(the equivalent of "P" in the first example.) Then the line "E-F" is extended and point "Y" is established by adding the length of "S-T" to "F-E" which takes us outside of the circle to "Y." The distance from "Y" to "X" (the equivalent of "P to Q" in the first example) when placed against the degree scale on the circle gave Wright "almost 97 degrees, of a great circle that is 1940 leagues or 5820 miles." the calculator gives 96.74 degrees or 5804 mile, a difference of 16 miles or 0.3% difference.
I don't know why it works and maybe somebody on the list can explain it to me. I spent some time with the law of cosines and found a way to reproduce this method using a calculator but you end up with a very long and inelegant formula.
BTW, it turns out that you never stop learning. I had always written the law of cosines as c= square root of (a ^2 + b ^2 - 2ab cos C) but working with it now I come up with c^2 = a^2 + b^2 -2ab cos C. I then saw the pattern for the first time, the first part looks just like Pythagoras' theorem. I then realized that the Pythagoras theorem is just a special case of the law of cosines. When C=90º its cosine becomes zero and the second part of the expression drops out leaving our old friend Pythagoras!
--~--~---------~--~----~------------~-------~--~----~
To post to this group, send email to NavList@fer3.com
To , send email to NavList-@fer3.com
-~----------~----~----~----~------~----~------~--~---
From: Gary LaPook
Date: 2007 Sep 21, 13:29 -0700
Edward Wright was a mathematician who turned his interest to navigation and was the first one to devise how to compute the table of meridional parts necessary to create charts on "Mercator's Projection" and published such a chart covering England to the Azores based on a voyage he took with the Earle of Cumberland in 1589. I attached a copy of that chart to a previous post. For biographical information on Wright you can go to: http://books.google.com/books?id=uTsJAAAAIAAJ&pg=PA101&lpg=PA101&dq=certaine+errors+in+navigation&source=web&ots=GqTcQV1VCi&sig=lyxvKOsPG1jE98_lxYLsaXprEiA#PPA101,M1
In "Certine Errors" Wright laid out a method to compute the great circle distance between two points on the earth using only a straight edge and a pair of dividers! I have never seen this method described in any other navigation text. Although it is now trivial to do this computation with a calculator it think it might be interesting to look at Wright's method. I am attaching 13 pages of his book that I photocopied back in 1980 but I am missing several pages which would fall between the second page of reproduced text (ending with "places.") and the next reproduced page starting with "foote," (they didn't number their pages.) Don't be intimidated by the type and spelling as you quickly get the hang of it and can read it as quickly as modern text. There also appears to be a couple of typographical errors that I will point out.
I will also offer my own explanation.
Wright starts out with special cases but I think it is easier if we start out with the general case. Wright's description of the general case starts on the last line of the third page of reproduced text ("If the latitudes be not both equall...") and continues with a couple of examples using London and Jerusalem ("Hierusalem") and London and Cusco as the examples. The coordinates he uses for London are 51º 32' north (which is as accurate as its modern latitude and a longitude of 22º (all of his longitudes are east). ( I do not know where he got this value since it does not comport with his own chart (which would make it about 16º) published in the same book, perhaps an earlier longitude using a different prime meridian (perhaps the Cape Verde Islands) since he couldn't be off by 6º between the Isle of Wright and London.) The latitude of Jerusalem he gives as 32º north while its modern value is 31º 16' north and he uses 11º south for Cusco while its correct value is 13º 28' south. The longitude he uses for Jerusalem is 68º, making it 46º east of London while its true value is 35º 14' east, a longitude error of 11º. He gives the longitude of Cusco as 295º making it 273º east of London while its modern longitude is 72º west, the same as 288º east of Greenwich showing an error of 15º between Wright's longitude and the modern value.
We will use Wright's values for this illustration, 51.5 for latitude of the London, 22 for the longitude of London; 32 and 68 for Jerusalem; and 11 south and 295 for Cusco.
Wright produces a diagram of the method however is is very complicated since all the lines for his many examples are contained on one diagram. We will draw only the lines we are interested in but will use Wright's labels for the lines.
Starting with the first example of figuring out the distance between London and Jerusalem, we first draw a line across the base circle diagram from the longitude of London, 22, "B" all the way across to the opposite side at 202., "C." Wright would have us next do the same for the longitude of Jerusalem but instead lets draw the line representing the latitude of London. Add its latitude, 51.5, to 22 and place a dot on the circle at 73.5, "E." Then taking a triangle or a plotter place its straight edge on the line "B-C" and draw a line from "E" that is perpendicular to "B-C," marking the intersection as "F." (Of interest is that the length of "E-F" is the sine of 51.5 and the length from the center of the circle (not labeled) to "F" is the cosine of 51.5.)
Next we do the same for the position of Jerusalem, drawing a line from 68, "M," across to 248, "J." Then we draw the perpendicular representing Jerusalem's latitude, from 100 (68+32) "N" to intercept "M-J" at "O." (The lengths of these lines represent the sine and cosine of 32.)
Since London and Jerusalem are both in the northern hemisphere, we next subtract the length of Jerusalem's latitude, "N-O," (the smaller value) from the length of the latitude of London, "E-F," by taking a pair of dividers and setting them to "N-O" and then placing one point on "E" and the other point on the line "E-F" and marking this point "Q." (Note, if you are following Wright's description at this point, there is a typo in the text were it states "and P Q equall to N O" it should say "and E Q equall to N O.") Next we determine the distance between the points "O" and "F" (the intersections of the latitude lines with their respective longitude lines) with our dividers and leaving one point on "F" we swing the other point to the line "B-C" and mark it "P." Leaving that point on "P" we next move the other point of the dividers to "Q," this is the end of the chase. With the dividers set to the spacing between "P" and "Q" we move the dividers to the scale on the circle and take out the number of degrees between the divider points, this is the number of degrees of the great circle joining the two places on earth. Multiply by 60 to calculate the number of nautical miles.
Does it work? Wright came up with "38 degrees and about 3/4 that is 775 leagues, which are 2325 miles." Using a modern digital calculator your end up with 38.614 degrees, 2316.8 miles a difference of 8.2 miles or a 0.3% difference. Not bad with just a straight edge and dividers.
Wright uses London and Cusco to illustrate the case when the two places are on opposite sides of the equator. The only difference is that instead of subtracting the latitude of one from the other you add them. You do this by extending the longer latitude line till it goes outside of the circle and then marking on this extended line the distance of the latitude of the other location. Using the diagram we already have and pretending that Jerusalem's latitude is south instead of north, we extend the line "E-F' some convenient distance outside the circle. Then placing the divider points on "N-O" we take off that spacing and placing one point on "E" we place the other point on the extended line outside the circle and this becomes our new "Q," lets call it "Q2." Then taking the distance from "Q2" to our original "P" we take out the spacing and place the dividers on the circle and find the number of degrees of the great circle between London and a Jerusalem located in the southern hemisphere. I come up with 92 degrees for a great circle distance of 5520 miles. Using a computer I get 5565 miles, a difference 45 miles or 0.8%.
Wright uses Cusco to illustrate this case, drawing in lines "R-V" and "S-T" in addition to the original lines for London. Taking the distance between "S" and "F" and using it to establish "X, "(the equivalent of "P" in the first example.) Then the line "E-F" is extended and point "Y" is established by adding the length of "S-T" to "F-E" which takes us outside of the circle to "Y." The distance from "Y" to "X" (the equivalent of "P to Q" in the first example) when placed against the degree scale on the circle gave Wright "almost 97 degrees, of a great circle that is 1940 leagues or 5820 miles." the calculator gives 96.74 degrees or 5804 mile, a difference of 16 miles or 0.3% difference.
I don't know why it works and maybe somebody on the list can explain it to me. I spent some time with the law of cosines and found a way to reproduce this method using a calculator but you end up with a very long and inelegant formula.
BTW, it turns out that you never stop learning. I had always written the law of cosines as c= square root of (a ^2 + b ^2 - 2ab cos C) but working with it now I come up with c^2 = a^2 + b^2 -2ab cos C. I then saw the pattern for the first time, the first part looks just like Pythagoras' theorem. I then realized that the Pythagoras theorem is just a special case of the law of cosines. When C=90º its cosine becomes zero and the second part of the expression drops out leaving our old friend Pythagoras!
--
warning
___
--~--~---------~--~----~------------~-------~--~----~
To post to this group, send email to NavList@fer3.com
To , send email to NavList-@fer3.com
-~----------~----~----~----~------~----~------~--~---